Mean values are not the natural object to study

Moments of the Riemann $\zeta$-function were introduced as a tool to attack the Lindelöff Hypothesis, which asserts that $\zeta(\frac12 + it)\ll t^\epsilon$ for any $\epsilon>0$. This is equivalent to

$$I_k(T)= \int_0^T \vert\zeta(\frac12 + it)\vert^{2k}dt\ll T^{1+\epsilon}$$

for any $\epsilon>0$, where the implied constant depends on $k$.

The above bound has only been established for $k=1$ and $2$, and for those values an asymptotic formula is known for the more general integral

$${\mathcal I}_k(a_1,...,a_{2k};w;T)= \int_{0}^\infty g(t/T)\prod_{j=1}^{2k} \zeta(\frac12 + a_j + \epsilon_j t)t^w\, dt,$$

where $\epsilon_j=\pm 1$ and $g$ is a function which descreases rapidly at $\infty$.

When $k=1$ or $2$, the function ${\mathcal I}_k$ can be continued to a meromorphic function. But if $k$ is larger, then ${\mathcal I}_k$ is (conjecturally) not continuable to a meromorphic function, and in fact it has a natural boundary. Diaconu, Goldfeld, and Hoffstein have shown that the function continues to a sufficiently large region that standard conjectures for the moments of $L$-functions should be able to be recovered from the polar divisors of ${\mathcal I}_k$. However, the fact that the function under consideration is not entire suggests that it may not be the correct object to study.

Problem: Find a natural way to modify ${\mathcal I}_k$ so that it becomes an entire (meromorphic) function.

The fact that ${\mathcal I}_k$ is not a nice function for $k\ge 3$ is an aspect of the ``Estermann Phenomonon.'' Consider the Dirichlet series

$$F_k(s)=\sum_{n=1}^\infty \frac{d(n)^k}{n^s},$$

where $d(n)=\sum_{ab=n} 1$ is the number of divisors of $n$. We have

$$\begin{eqnarray*} F_0(s)&&=1\cr F_1(s)&&=\zeta(s)^2\cr F_2(s)&&=\frac{\zeta(s)^4}{\zeta(2s)} \cr \end{eqnarray*}$$

But if $k\ge 3$ then $F_k(s)$ can only be expressed as an infinite product of $\zeta$-functions, and it has a natural boundary at $\sigma = 0$.

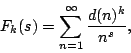
An example which may be more closely related to the situation at hand is

$$F(s)=\sum_{n=1}^\infty \frac{d_3(n)^2}{n^s},$$

where $d_3(n)=\sum_{abc=n} 1$. Again $F(s)$ has a natural boundary at $\sigma = 0$, but is is possible to modify the coefficients of $F(s)$, only involving the terms divisible by a cube, so that the function has an analytic continuation. This is promising because a partial sum of $F(s)$ is the diagonal contribution to the integrand of $I_3(T)$.

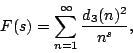
See Titchmarsh [ MR 88c:11049] for more background information.

Back to the main index for L-functions and Random Matrix Theory.